Cartesian Product Of Intervals . Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals Given two sets a and b, it is possible to “multiply” them to produce a new set denoted as a × b. A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. The cartesian product of a and b is the set. Web since the cartesian product \(\mathbb{r} ^2\) corresponds to the cartesian plane, the cartesian product of two subsets. B\in b\}$$ so in this case it would consist of all the points (coordinates in. Web i know what is a cartesian product of sets, for example, $m= \{1,2\} , n = \{a,b\} $ $m \times n = {(1,a), (1,b), (2,a) , (2,b)}$ but what is the cartesian product of two. Web the cartesian product is defined as $$a\times b:=\{(a,b)\mid a\in a,\;
from www.numerade.com
Given two sets a and b, it is possible to “multiply” them to produce a new set denoted as a × b. Web since the cartesian product \(\mathbb{r} ^2\) corresponds to the cartesian plane, the cartesian product of two subsets. The cartesian product of a and b is the set. A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. B\in b\}$$ so in this case it would consist of all the points (coordinates in. Web the cartesian product is defined as $$a\times b:=\{(a,b)\mid a\in a,\; Web i know what is a cartesian product of sets, for example, $m= \{1,2\} , n = \{a,b\} $ $m \times n = {(1,a), (1,b), (2,a) , (2,b)}$ but what is the cartesian product of two. Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals
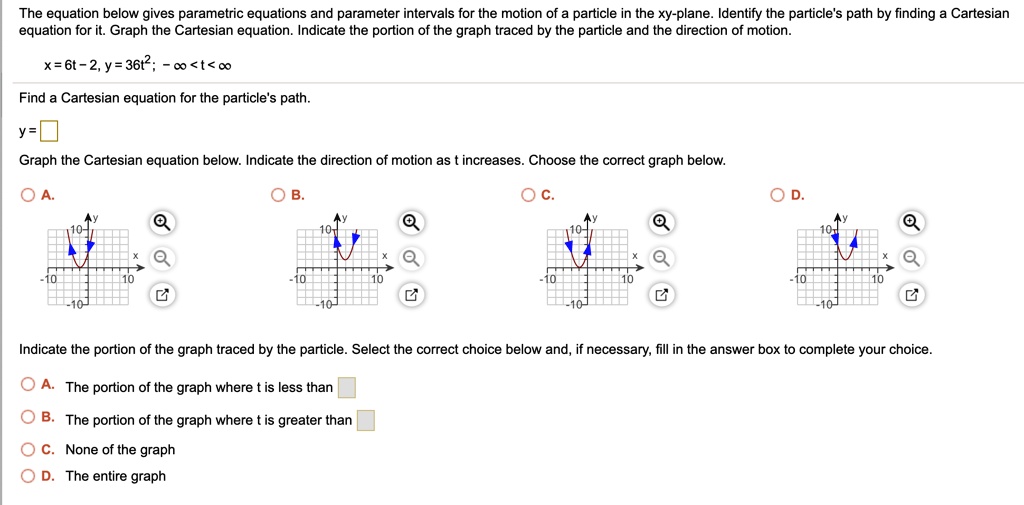
SOLVED The equation below gives parametric equations and parameter
Cartesian Product Of Intervals Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals Web the cartesian product is defined as $$a\times b:=\{(a,b)\mid a\in a,\; Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals Given two sets a and b, it is possible to “multiply” them to produce a new set denoted as a × b. A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. B\in b\}$$ so in this case it would consist of all the points (coordinates in. Web since the cartesian product \(\mathbb{r} ^2\) corresponds to the cartesian plane, the cartesian product of two subsets. The cartesian product of a and b is the set. Web i know what is a cartesian product of sets, for example, $m= \{1,2\} , n = \{a,b\} $ $m \times n = {(1,a), (1,b), (2,a) , (2,b)}$ but what is the cartesian product of two.
From math.stackexchange.com
If two graphs are planar, will the cartesian product between them be Cartesian Product Of Intervals Web the cartesian product is defined as $$a\times b:=\{(a,b)\mid a\in a,\; Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals The cartesian product of a and b is the set. B\in b\}$$ so in this case it would consist of all the points (coordinates in. Web i know what is a cartesian product of. Cartesian Product Of Intervals.
From www.chegg.com
Solved 1. Set × Cartesian × Product × Proof [16 Points ] Cartesian Product Of Intervals Web i know what is a cartesian product of sets, for example, $m= \{1,2\} , n = \{a,b\} $ $m \times n = {(1,a), (1,b), (2,a) , (2,b)}$ but what is the cartesian product of two. A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. B\in b\}$$ so in this case. Cartesian Product Of Intervals.
From www.youtube.com
How to represent Cartesian product by using arrow Diagram YouTube Cartesian Product Of Intervals Given two sets a and b, it is possible to “multiply” them to produce a new set denoted as a × b. Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. The cartesian product of a. Cartesian Product Of Intervals.
From www.mdpi.com
Mathematics Free FullText The Diagnosability of the Generalized Cartesian Product Of Intervals B\in b\}$$ so in this case it would consist of all the points (coordinates in. Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals Web since the cartesian product \(\mathbb{r} ^2\) corresponds to the cartesian plane, the cartesian product of two subsets. Web the cartesian product is defined as $$a\times b:=\{(a,b)\mid a\in a,\; Web. Cartesian Product Of Intervals.
From www.nagwa.com
Question Video Finding the Union of Two Cartesian Products of Given Cartesian Product Of Intervals Web since the cartesian product \(\mathbb{r} ^2\) corresponds to the cartesian plane, the cartesian product of two subsets. Web the cartesian product is defined as $$a\times b:=\{(a,b)\mid a\in a,\; A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. Given two sets a and b, it is possible to “multiply” them to. Cartesian Product Of Intervals.
From www.researchgate.net
(PDF) Fuzzy Incidence Coloring on cartesian product of some Fuzzy Cartesian Product Of Intervals Web since the cartesian product \(\mathbb{r} ^2\) corresponds to the cartesian plane, the cartesian product of two subsets. Web i know what is a cartesian product of sets, for example, $m= \{1,2\} , n = \{a,b\} $ $m \times n = {(1,a), (1,b), (2,a) , (2,b)}$ but what is the cartesian product of two. Given two sets a and b,. Cartesian Product Of Intervals.
From www.numerade.com
SOLVED Problem Suppose A and B are denumerable sets Prove that the Cartesian Product Of Intervals B\in b\}$$ so in this case it would consist of all the points (coordinates in. Web since the cartesian product \(\mathbb{r} ^2\) corresponds to the cartesian plane, the cartesian product of two subsets. A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. Web #cartesian_product #intervalsin this topic, students learn how to. Cartesian Product Of Intervals.
From www.numerade.com
SOLVED The equation below gives parametric equations and parameter Cartesian Product Of Intervals Web since the cartesian product \(\mathbb{r} ^2\) corresponds to the cartesian plane, the cartesian product of two subsets. A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals Web i know what is a cartesian product of. Cartesian Product Of Intervals.
From www.alamy.com
Cartesian coordinate system template. Perpendicular X and Y axises Cartesian Product Of Intervals Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals B\in b\}$$ so in this case it would consist of all the points (coordinates in. Web i know what is a cartesian product of sets, for example, $m= \{1,2\} , n = \{a,b\} $ $m \times n = {(1,a), (1,b), (2,a) , (2,b)}$ but what. Cartesian Product Of Intervals.
From slideplayer.com
Sets Section ppt download Cartesian Product Of Intervals B\in b\}$$ so in this case it would consist of all the points (coordinates in. Web i know what is a cartesian product of sets, for example, $m= \{1,2\} , n = \{a,b\} $ $m \times n = {(1,a), (1,b), (2,a) , (2,b)}$ but what is the cartesian product of two. A × b = {(a, b) ∣ a ∈. Cartesian Product Of Intervals.
From mathoriginal.com
Cartesian product and Relation of two sets Math Original Cartesian Product Of Intervals Web the cartesian product is defined as $$a\times b:=\{(a,b)\mid a\in a,\; The cartesian product of a and b is the set. Web since the cartesian product \(\mathbb{r} ^2\) corresponds to the cartesian plane, the cartesian product of two subsets. Given two sets a and b, it is possible to “multiply” them to produce a new set denoted as a ×. Cartesian Product Of Intervals.
From www.youtube.com
Algebra 11 Cartesian Coordinates in Three Dimensions YouTube Cartesian Product Of Intervals Given two sets a and b, it is possible to “multiply” them to produce a new set denoted as a × b. Web the cartesian product is defined as $$a\times b:=\{(a,b)\mid a\in a,\; B\in b\}$$ so in this case it would consist of all the points (coordinates in. Web i know what is a cartesian product of sets, for example,. Cartesian Product Of Intervals.
From courses.cs.washington.edu
Cartesian Product Cartesian Product Of Intervals Web i know what is a cartesian product of sets, for example, $m= \{1,2\} , n = \{a,b\} $ $m \times n = {(1,a), (1,b), (2,a) , (2,b)}$ but what is the cartesian product of two. A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. Given two sets a and b,. Cartesian Product Of Intervals.
From www.researchgate.net
9 Cartesian product of two graphs. Download Scientific Diagram Cartesian Product Of Intervals Web since the cartesian product \(\mathbb{r} ^2\) corresponds to the cartesian plane, the cartesian product of two subsets. A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals Web the cartesian product is defined as $$a\times b:=\{(a,b)\mid. Cartesian Product Of Intervals.
From www.aimspress.com
Completely independent spanning trees in some Cartesian product graphs Cartesian Product Of Intervals Given two sets a and b, it is possible to “multiply” them to produce a new set denoted as a × b. Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. B\in b\}$$ so in this. Cartesian Product Of Intervals.
From www.flexiprep.com
NCERT Class 11 Mathematics Solutions Chapter 2 Relations and Cartesian Product Of Intervals Web i know what is a cartesian product of sets, for example, $m= \{1,2\} , n = \{a,b\} $ $m \times n = {(1,a), (1,b), (2,a) , (2,b)}$ but what is the cartesian product of two. Web the cartesian product is defined as $$a\times b:=\{(a,b)\mid a\in a,\; Web since the cartesian product \(\mathbb{r} ^2\) corresponds to the cartesian plane, the. Cartesian Product Of Intervals.
From www.youtube.com
How to represent Cartesian product by using Cartesian Diagram YouTube Cartesian Product Of Intervals Web #cartesian_product #intervalsin this topic, students learn how to find cartesian product of two intervals The cartesian product of a and b is the set. Web the cartesian product is defined as $$a\times b:=\{(a,b)\mid a\in a,\; A × b = {(a, b) ∣ a ∈ a ∧ b ∈ b} (4.4.1) thus, a ×. Web i know what is a. Cartesian Product Of Intervals.
From www.youtube.com
Cartesian Product of Two Sets Relations & Functions Chapter 1 (L1 Cartesian Product Of Intervals The cartesian product of a and b is the set. Web i know what is a cartesian product of sets, for example, $m= \{1,2\} , n = \{a,b\} $ $m \times n = {(1,a), (1,b), (2,a) , (2,b)}$ but what is the cartesian product of two. Given two sets a and b, it is possible to “multiply” them to produce. Cartesian Product Of Intervals.